Geodesics
Active in several areas of the COVID-19 pandemic, the Global Publications department at Global Youth Group has always shared accurate and relevant information regarding the coronavirus pandemic and its impact on us today, globally. This article, however, is different from our other articles; it will highlight the interests of math, physics, and non-Euclidean geometry as it explains the concepts of “geodesics”.

BY KARANVEER SINGH
You must be familiar with the idea that the shortest distance between two points is a straight line. It is one of the fundamentals of Euclidean geometry and can be proved using the calculus of variation or the principle of least action. But there is an important assumption: the surface is flat.
Let us assume a sphere (it has a non-flat surface). If the sphere is large enough, it can provide a flat surface at tiny distances. Keeping this in mind, we mark two points on the opposite sides of the sphere. What is the shortest possible distance between those two points? If your answer is “the same as the diameter of the sphere.”, that is right. Now, let me reiterate my question. What is the shortest possible distance between those two points if you were restricted to move along the surface of the sphere? The curved line joining the two points would have a length of ΠR and is called a geodesic.
The idea of a curved line being the shortest distance between two points is not one from Euclidean geometry. Hence, some rules of Euclidean geometry are no longer valid.
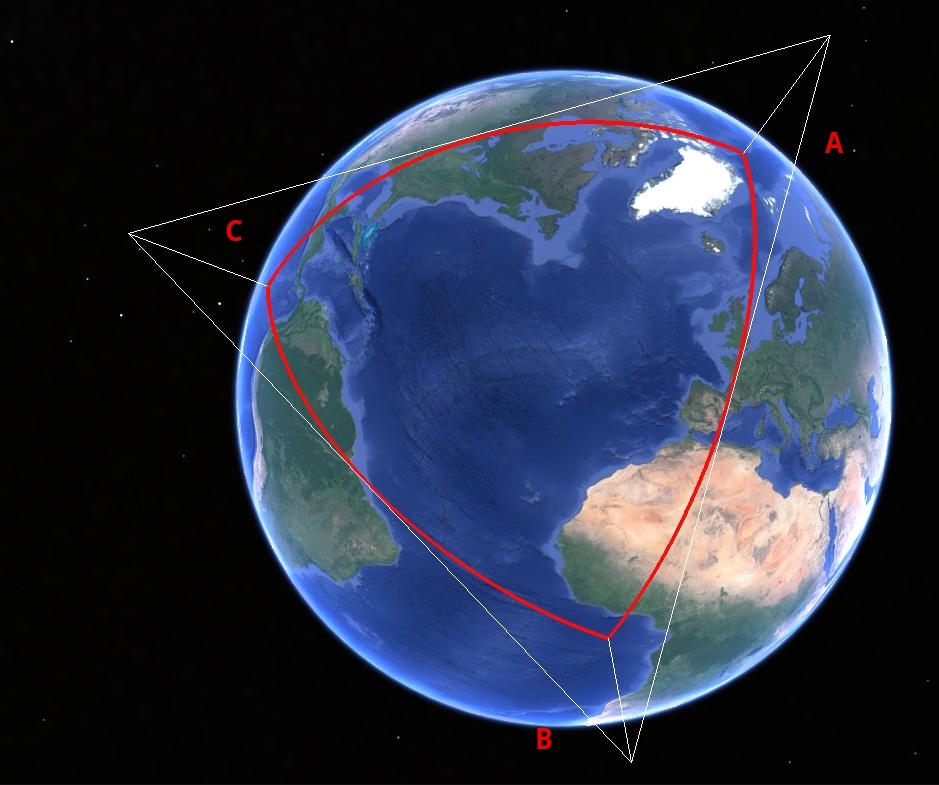
Consider now the triangle on the surface of the Earth made up of the Equator, the line of 0 degrees longitude, the line of 90 degrees longitude east. Both of the longitude lines make an angle of 90 degrees with the Equator. The two lines also meet each other at the North pole at an angle of 90 degrees. The angles of this triangle add up to 270 degrees. Hence, the statement ‘Euclidean geometry is only good for flat surfaces’ is right because 270 is not equal to 180.
According to Einstein’s theory of General relativity, matter bends space-time (4-dimensional fabric of the universe), and the curvature of space-time influences the movement of matter. Geodesics can, simply, be defined as the path taken by light. This association of light with geodesics was key in proving Einstein’s General Relativity right. On a flat surface, light moves in a straight line, but as the surface bends, the path of the light curves. On May 29 1919, this idea was tested. Arthur Eddington and his team proved that the stars’ apparent positions moved during the eclipse. This was the first time General Relativity was tested and proven.
References
- Taimina, D. and Henderson, . David W. (2020, September 9). Non-Euclidean geometry. Encyclopedia Britannica. https://www.britannica.com/science/non-Euclidean-geometry
